How You Can Easily Find GCF? A Step by Step Solution

The term greatest common factor (GCF), highest common factor (HCF), and
Greatest common divisor (GCD) refer to the same thing.
What is GCF?
By definition GCF means:
“The greatest common factor is the greatest number which is a common
factor of any two numbers under consideration.”
It is not necessary that common factors exist only among the factors of “two”
numbers. There is not as such any limit. You can look for GCF among 3, 6, or
even 10.
Before we have a deeper look at the greatest common factor and how to find the
greatest common factor, let’s first see what is a factor?
What is a factor?
“Such a number which can divide the given number to give 0 as a reminder.”
OR
“The numbers which have the under discussion number in their tables are called factors of that number.”
An easy way to learn factors is by examples:
We take the number 15. To find its factors we need to know in which tables it is present.
- As “1” has every number in its table so the first factor is one.
- After that 15 is on the table of 3 and 15 only.
So the total factors for number fifteen are 3. We write it as:
Factors of 15: 1,3,15
Factors of some other numbers are:
| Factors of 20 | 1, 2, 4, 5, 10, 20 |
| Factors of 35 | 1, 5, 7, 35 |
| Factors of 29 | 1, 29 |
| Factors of 60 | 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, 60 |
| Factors of 54 | 1, 2, 3, 6, 9, 18, 27, 54 |
How to find GCF?
There are a number of ways you can find the GCF of numbers. There are also online calculators available to find GCF. We learn how to find the greatest common factor through different methods step-by-step.
Through online calculator:
If you don’t know how to find GCF and don’t have time to learn, then an easy way is to use the GCF calculator. This calculator can find the answer by using the method of your choice.
It has 5 different options to choose from. Once you put the numbers, separated by commas, it will solve them and give you a step-by-step solution with an accurate answer.
By Factorization method:
One of the easiest ways to find GCF is through the factorization method. Here is a step-by-step guide with an example.
Example 1:
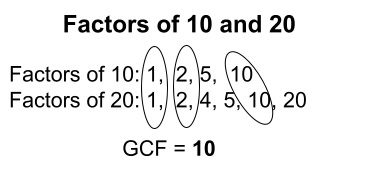
Find the greatest common factor of 20 and 10
Step 1: find the factors of all the numbers.
Step 2: Draw circles around the common factors of both numbers.
Step 3: choose the highest number. This number is GCF.
Example 2:
Find the GCF of 24 and 76 by factorization method.
Solution:
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Factors of 76: 1, 2, 4, 19, 38, 76
Common factors are 1,2 and 4. Since 4 is the greatest one so it is GCF.
By prime Factorization:
In this method, we make a factor tree to identify the highest or greatest common factor.
Example 3:
Find the GCF of 35 and 45.
Step 1: First we make a factor tree that is very similar to finding LCM.
Step 2: Encircle the common factors.
Step 3: Multiply the common numbers and the result you will get is your greatest common factor.
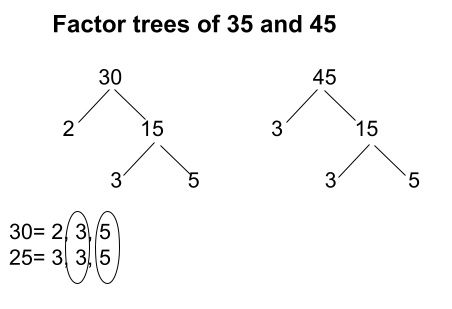
GCF = 3 5 = 15
Example 4:
What is the GCF of 22 and 48? Solve by prime factorization.
24 , 48
2 12 2 24
2 6 2 12
2 3 2 6
2 3
22= 2, 2, 2, 3
48= 2, 2, 2, 2, 3
GCF = 2 22 3= 24
By Division method:
Another manual method to find the greatest common factor is through this method. This one is tricky.
Example 5:
Find the highest common factor of 30 and 14 through the division method.
Step 1: Divide the higher value by the lower value.

In our example 14 was a lower value. After dividing we have a remainder 2.
Step 2: Now, take the remainder of the first step on the outside and divide the lower value, divisor of the first step, by it. Keep repeating this process until you get 0 as a remainder.
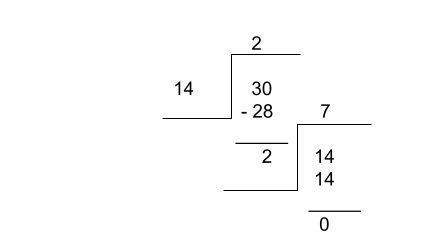
In our example, we had to do only two steps.
Step 3: the last value dividing by which the remainder was zero, is the HCF. In the above-mentioned example, 2 is the HCF or the greatest common factor.
Example 6:
By the Division method, find the GCF/HCF of 16 and 54.
Solution:
As 16 is the smaller number
1. By dividing 54 by 16, we get 6 as the remainder.
2. By dividing 16 by 6, we get 4 as the remainder.
3. By dividing 6 by 4, we get 2 as the remainder.
4. By dividing 4 by 2, we get 0 as the remainder.
So GCF is 2.
By upside down division method:
The name “Upside down division” is given to this method because in this method we place the division bar inverted.
Example 4:
Find the GCF of 30 and 65 by the upside-down method.
Step 1: place all the numbers side by side in an upside-down bar.Step 2: choose a number that can divide your numbers and start this in ascending process. First, try 2 then 3 then 4, and so on
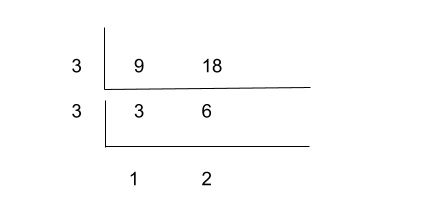
Step 3: Repeat the process until no more division is possible.
Step 4: Multiply the numbers on the side, as in our case we have 3 and 3, to find GCF.
In our example, GCF is 9.